Vc = known V-mag for calibration star,
Rc = known R-mag for calibration star,
Sv = V-band star color sensitivity (slope of plot: V-mag error versus star color C, for several calibrated stars), explained more elsewhere,
Sr = R-band star color sensitivity (slope of plot: R-mag error versus star color C, for several calibrated stars), explained more elsewhere,
C = star color, defined to be V - R - 0.4,
Ct = star color of target star,
Cc = star color of calibration star,
Vt = V-mag of target star,
Rt = R-mag of target star,
vi = instrumental magnitude = -2.5 × LOG10 (Fi / g) + 15 (15 is a zero-shift constant that doesn't matter),
Fi = flux of star i,
g = exposure time
IMAGES AND CONVERTING FLUX MEASUREMENTS TO STANDARD MAGNITUDES
The Landolt star field that I refer to as L0652 has many calibrated stars that fit within my FOV. Here's an image that I'll use to illustrate differential photometry concepts.
Figure. 1. Landolt area at 06:52:08 -00:19:42 (FOV center). FOV = 16 x 11 'arc. North up, east left. Stars "c" and "t" will be used in as the calibration and target stars.
Star "c" has Vc = 8.378, Rc = 8.307. A Celestron 14-inch telescope was used to observe this star field with V and Rc filters. Exposures were 10 seconds.
Here are the manually read star fluxes for V and R images:
Fcv = 260974, Fcr = 371763, Ftv = 91557, Ftr = 120527.
These fluxes correspond to instrumental magnitudes of :
vc = 15 -2.5× LOG10 (260974 / 10) = 3.959
vt = 15 -2.5× LOG10 ( 91557 / 10) = 5.096
rc = 15 -2.5× LOG10 (371763 / 10) = 3.574
rt = 15 -2.5× LOG10 ( 120527 / 10) = 4.797
Cc = 8.378 - 8.307 - 0.4
Cc = -0.329
Ct = { (8.378 - 8.307) × ( 1 + (-0.07) - (-0.07)) + (5.096 - 4.797) - (3.959 - 3.574) } / { 1 + (-0.10) - (-0.07) } - 0.4
Ct = -0.42
Vt = 8.378 + (5.096 - 3.959) + (-0.07) × ((-0.42) - (-0.33))
Vt = 9.52
Rt = 8.307 + (4.797 - 3.574) + (-0.10) × ((-0.42 - (-0.33))
Rt = 9.54
All these calculations are easily performed by a spreadsheet, where only 8 cells require user entry. Here's an example.
These fluxes correspond to instrumental magnitudes of :
vc = 15 -2.5× LOG10 (260974 / 10) = 3.959
vt = 15 -2.5× LOG10 ( 91557 / 10) = 5.096
rc = 15 -2.5× LOG10 (371763 / 10) = 3.574
rt = 15 -2.5× LOG10 ( 120527 / 10) = 4.797
Cc = 8.378 - 8.307 - 0.4
Cc = -0.329
Ct = { (8.378 - 8.307) × ( 1 + (-0.07) - (-0.07)) + (5.096 - 4.797) - (3.959 - 3.574) } / { 1 + (-0.10) - (-0.07) } - 0.4
Ct = -0.42
Vt = 8.378 + (5.096 - 3.959) + (-0.07) × ((-0.42) - (-0.33))
Vt = 9.52
Rt = 8.307 + (4.797 - 3.574) + (-0.10) × ((-0.42 - (-0.33))
Rt = 9.54
All these calculations are easily performed by a spreadsheet, where only 8 cells require user entry. Here's an example.
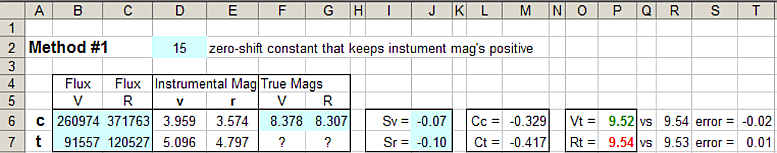
Figure 2. Spreadsheet showing manually read star fluxes for V and R images, their corresponding "instrument magnitudes," known magnitudes for the calibration star, and calculations corresponding to the procedure described in the previous section. The light blue cells are for user input.
The suggested procedure yields Vt = 9.52 and Rt = 9.54. The target star is actually one of the stars in the Landolt list, so we can compare the magnitudes calculated using my suggested procedure and Landolt's magnitudes. The "errors" using my procedure are -0.02 and +0.01 magnitude. This is an adequate result.
Return to calling web page: link