Introduction

HD 80606 has an exoplanet that orbits with a 111-day period in a very eccentric orbit (0.93). The orbit is inclined close to 90 degrees because when it is behind the star it undergoes a "secondary" transit, as observed with the Spitzer Space Telescope by Laughlin et al (2009). It is not known if the planet transits in front of the star, for a primary transit, because that part of its orbit is much farther from the star. An opportunity for observing a possible primary transit exists on Feb 13/14 UT of 2009. This web page has been created for offering suggestions on the best strategy for conducting these observations and for processing the images to produce a light curve. The general case (no nearby interfering star) is described at DefocusingGeneralCase.

Hardware

My telescope is a 11-inch aperture Celestron (CPC1100) mounted on an equatorial wedge. I use a focal reducer and SBIG AO-7 image stabilizer in front of a SBIG ST-8XE CCD camera. The AO-7 employs a tip/tilt mirror that can be operated at several Hz, and when mirror motion exceeds a user-specified limit a correcting nudge signal is sent to the telescope drive motors. The polar axis has been aligned to the north celestial pole with an accuracy of ~2 'arc. This assures that when the AO-7 keeps an autoguided star fixed to a pixel location on the autoguider chip the star field does not rotate about that star and simultaneously move the star field across the main CCD chip. This arrangement assures that the star field is fixed with respect to the CCD pixel field to within a few pixels during the course of a many hour observing session. The image scale is 1.21 "arc/pixel, and the FOV is 31 x 21 'arc. I use a wireless focuser (Craycroft type) for adjusting the position of the optical back-end (the mirror is not moved). MaxIm DL 5.03 is used to control the telescope, wireless focuser, AO-7 and CCD camera.

Each observer's hardware is different, and atmospheric seeing is different each night, so the following is presented with the purpose of illustrating concepts that can be used to guide observers in choosing optimum observing and image analysis strategies.

Review of Usual Strategy for Exoplanet Transit Light Curves

Normally, transiting exoplanet light curves are produced using sharply-focused images and a photometry aperture radius ~ 4 x FWHM. Slightly smaller and larger photometry apertures can sometimes produce small improvements (e.g., ApertureRadius/FWHM within range 2 to 6). However, when a star is close to the target star it may not be possbile to employ this standard aperture setting. Exposure times are chosen that yield maximum counts for stars of interest that are slightly less than the linearity limit (52,000 counts for my CCD) at times of best atmospheric seeing. Here's an image of the HD 80606 star field.

Figure 1. Star field surrounding HD 80606 (indicated) and it's orbital companion star, HD 80607 (to its left). FOV = 31 x 21 'arc, north up, east left. FWHM ~ 4.2 "arc.

Figure 2. Zoom of previous image showing a photometry aperture centered on HD 80606 and HD 80607 20.5 "arc to the east. FOV = 9.3 x 8.2 'arc. The aperture radius is 9.7 "arc (8 pixels), which is ~2 x FWHM for most images of this observing session.

Fig. 2 gives the impression that it is possible to employ a small photometry aperture for stars as close as 20.5 "arc (17 pixels). However, the brightness/contrast setting for this image gives a false impression of each star's PSF (point-spread function). Here's a different brightness/contrast choice.

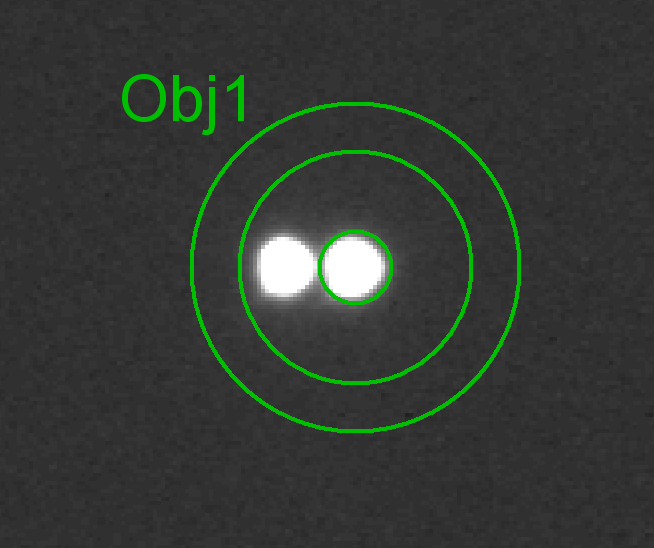
Figure 3.  Zoom showing a photometry aperture radius of 10.9 "arc (9 pixels), which places a boundary of the signal aperture half-way between the two stars.

Fig. 3 shows a signal aperture choice that should be least affected by atmospheric seeing changes that cause the PSF of the nearby star, HD 80607, to "spill over" into the signal aperture while the same seeing change causes the target star's PSF to "spill out" of the signal aperture by approximately the same amount. For this observing session, and for exposure times of 30 seconds (the longest exposure time that still prevents saturation), PSFs have FWHM values that are typically 4.5 "arc. This aperture corresponds to the ratio ApertureRadius /  FWHM  = 2.0, which is not within the customary 3 to 5 range where best results are usually obtained. This suggests that using this aperture it may not be possible to obtain a high precision light curve.

Three Strategy Candidates

In anticipation of problems with the usual observing strategies I note three approaches to observing HD 80606:

    1) Choose a photometry aperture radius of 1/2 the separation of HD 80606 and HD 80607 (i.e., 1/2 of 20.5 "arc ~ 10 "arc), and hope this is adequate (e.g., Fig.3),
    2) Choose a photometry aperture that includes both stars, and accept the added noise from the larger number of pixels within the signal aperture (e.g., Fig. 8, below), and
    3) Defocus all images the same amount and use a large photometry aperture to include both stars (Fig. 10, below).

Note that a defocused image can accomodate a much longer exposure time than a sharply focused image, thus improving the duty cycle (defined as "time exposing an image divided by interval between images"). For example, one of my image sets had an exposure time of 10 seconds (sharply focused) and the defocused images had an exposure itme of 60 seconds. Since my overhead is 12 seconds (8 seconds for image download and 4 seconds for the AO-7 to adjust tracking), the corresponding duty cycles are 45% and 83%. The number of photons collected per unit time are in the same ratio, 45 and 83, so the defocused image set yields almost twice as many photons per unit observing time as the 10-second image set. Duty cycle advantages for defocusing are greater for bright stars since brightness calls for short exposure times for sharply focused images (to avoid saturation).

Strategy 1: Sharp focus, small aperture

This strategy is as close to what normally is done as possible; only the photometry aperture is smaller than normal because of the nearby star HD 80607. The exposure time was 30 seconds, which kept all stars in the FOV just below saturation. The following image shows my choice for reference stars.

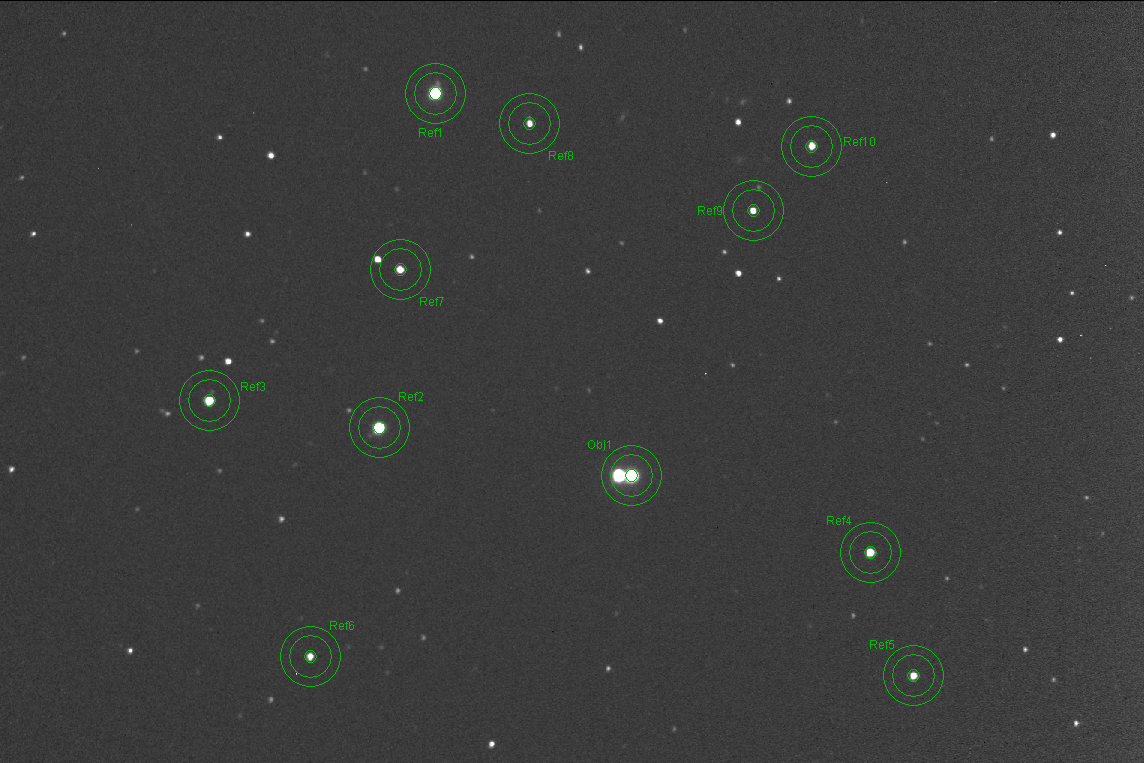
Figure 4. Sharply focused and 30-second exposure star field with 10 reference stars indicated.

This strategy was employed for an hour, and the next figure shows processed light curve.

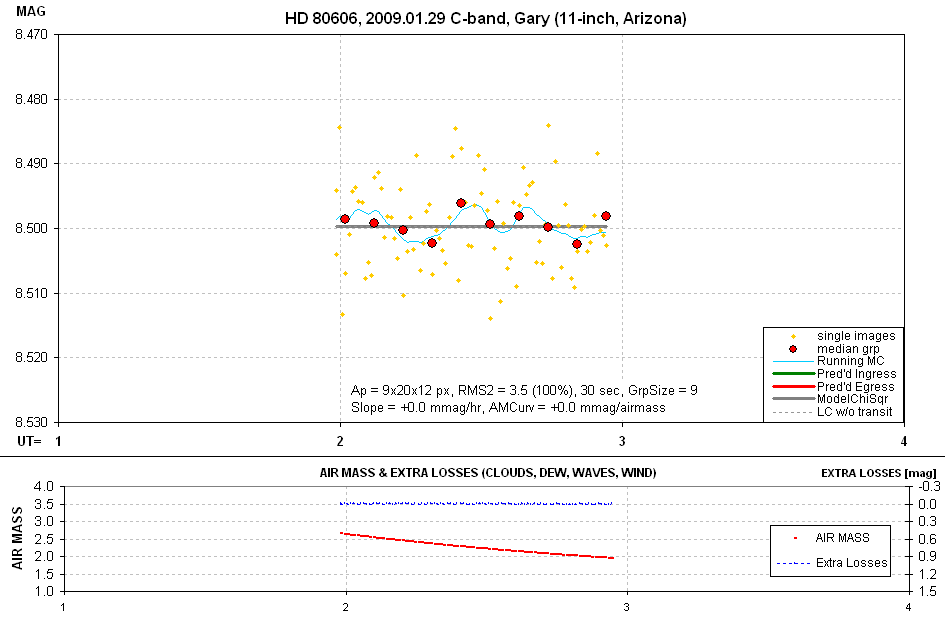
Figure 5. Light curve of HD 80606 for the sharply focused, 30-second exposure image set.

The single image RMS is equivalent to a normalized 2-minute exposure having RMS = 3.5 mmag per image. Taking the 71% duty cycle into account these data correspond to achieving a precision of 4.15 mmag per 2 minutes of observing time. This is greater than I normally achieve (edven for 11th magnitude stars), so this appears to support my expectation that the precision suffers from having to use ApertureRadius/FWHM = 2 instead of the normally optimum 4.

It occured to me that I could improve precision if shaper images could be achieved. One way to improve sharpness is to use shorter exposures. So I took another hour of 10-second exposures. 

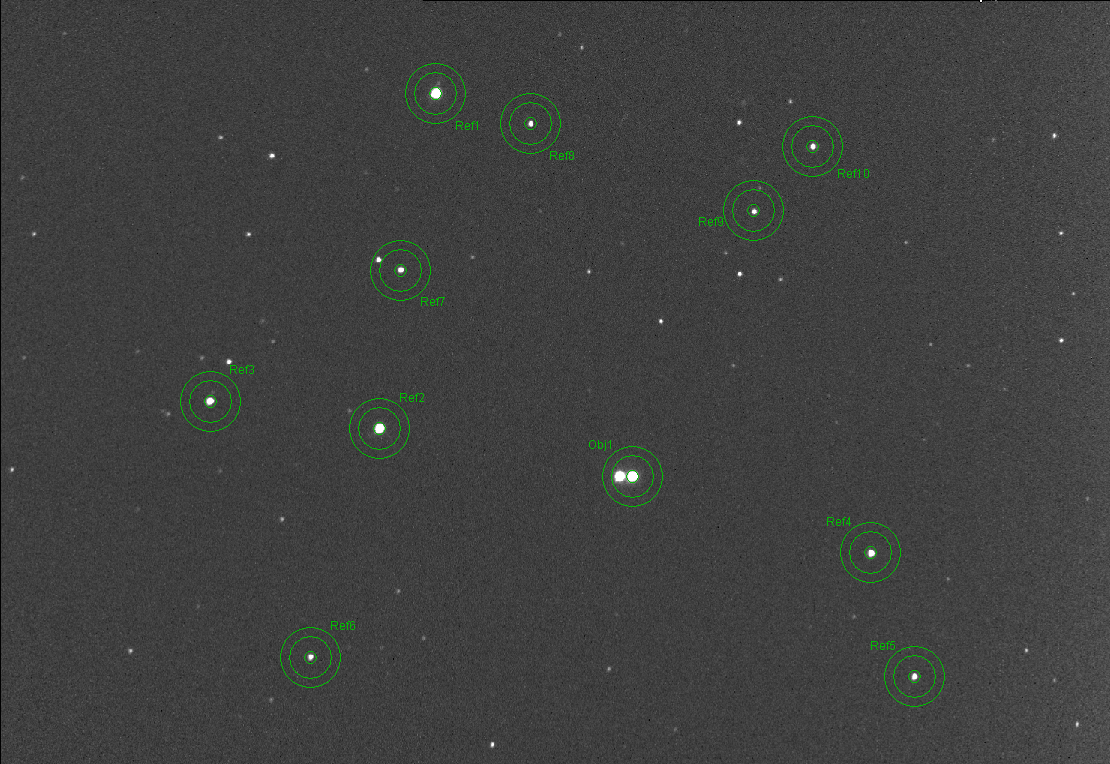
Figure 6. Shorter exposure image (10 seconds instead of 30 seconds) showing the same photometry aperture setting as before (9x20x12 pixels). 

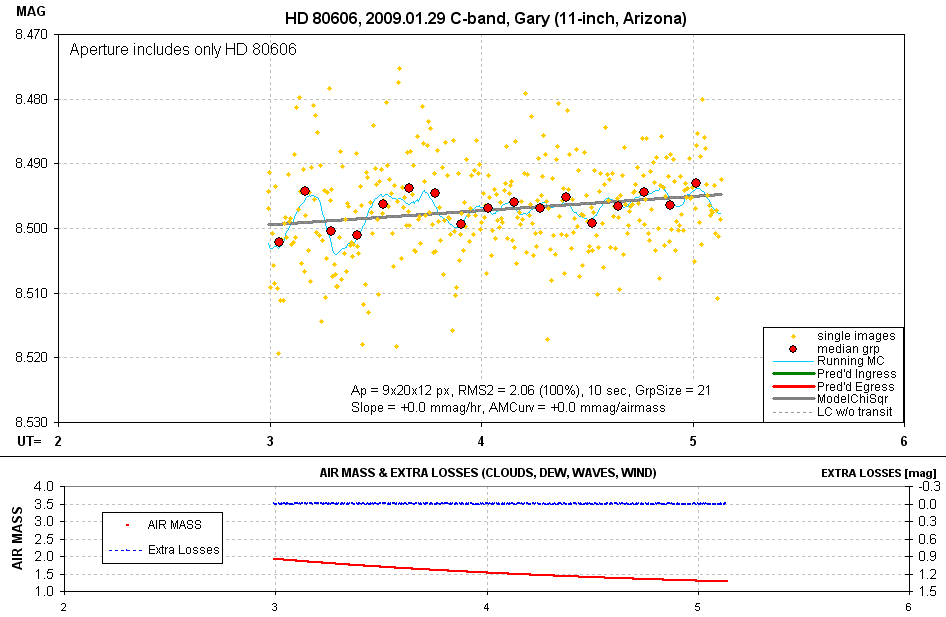
Figure 7. Light curve for above set of images.

The precision per image, normalized to a 2-minute exposure, is 2.06 mmag, which is the kind of improvement that what I was hoping for. However, 10-second imaging has a duty cycle of 45%, and adjusting for this yields a precision of 3.07 mmag per 2 minutes of observing time. This is still significantly better than the precision of the 4.15 mmag per 2 minutes of observing time calculated from the 30-second exposure image set.

Strategy 2: Sharp focus, large aperture

The 10-second images were processed using an aperture that included the flux fromboth HD 80606 and HD 80607, as shown in the next image.

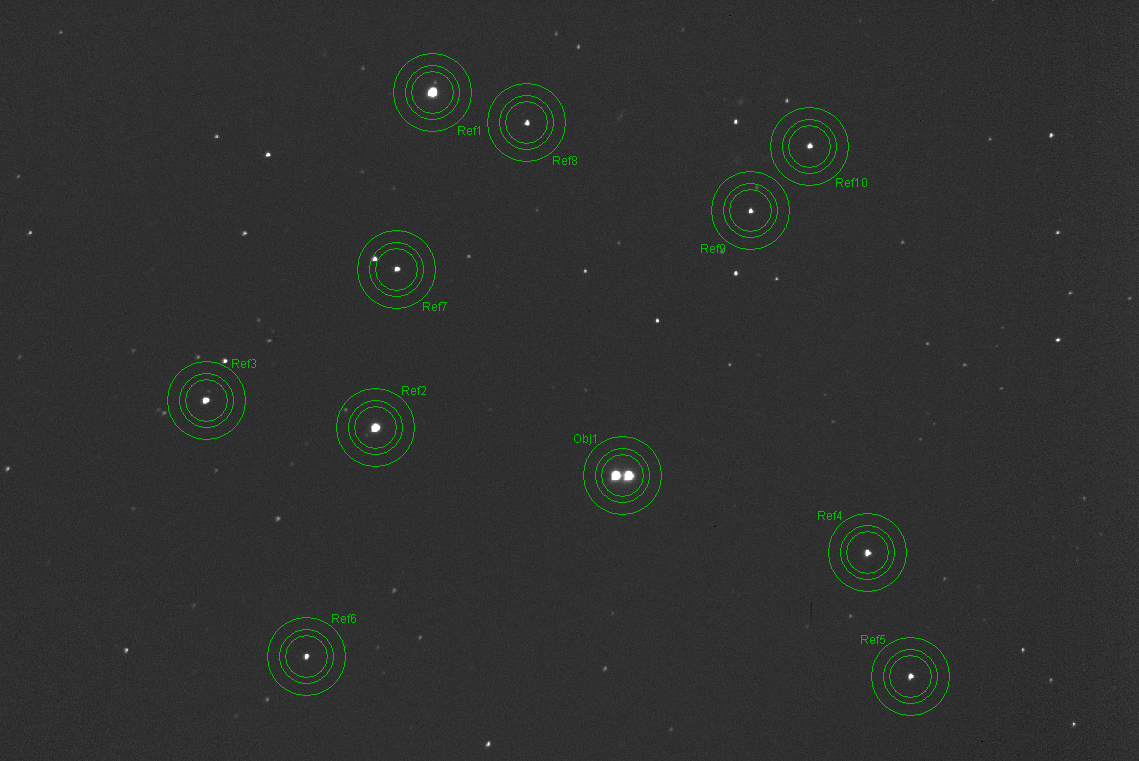
Figure 8. Using a large aperture for the same images as in the previous analysis (10-second exposures). Aperture dimensions are 28x8x16 pixels.

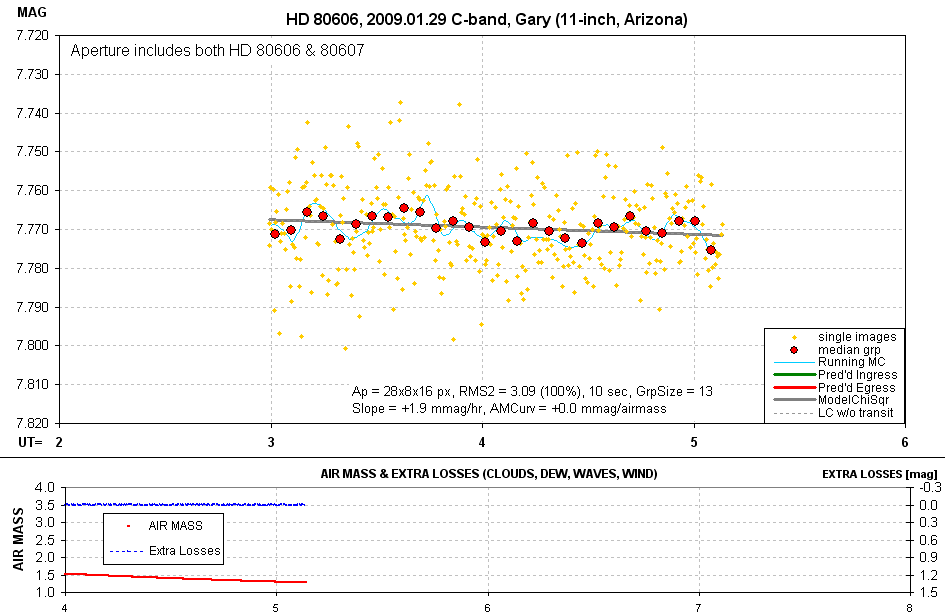
Figure 9. Light curve from 10-second, sharply focused images but using a large photometry aperture that includes both stars. 

The large aperture led to a worsened RMS per image. After correcting for duty cycle the precision is 4.61 mmag per 2-minutes of observing time.

Strategy 3: Defocused, large aperture

The following defocused image is from a 1-hour observing session immediately following the previous image set.

Figure 10. Defocused image showing the same large photometry aperture used with the sharply focused images. Exposure time is 60 seconds.

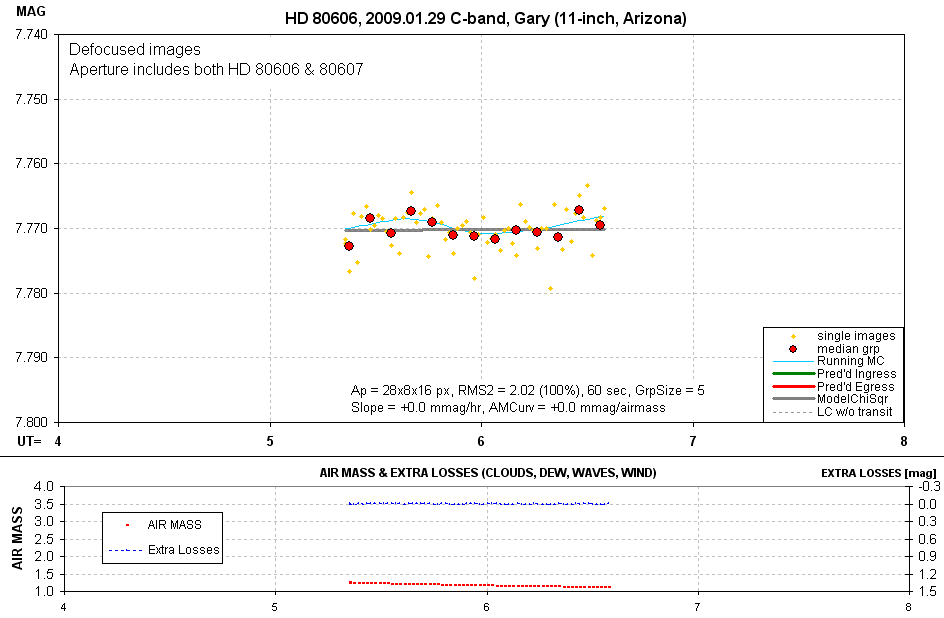
Figure 11. Light curve from defocused images and large photometry aperture that included both stars.

The 2-minute equivalent RMS per image is 2.02 mmag, and correcting for duty cycle yields 2.22 mmag per 2 minutes of observing time. This is a smaller RMS than for any of the other observing and analysis strategies. However...

Final Assessment

The best RMS per image was obtained using a defocused strategy. However, recall that when two equally bright stars are included in the photometry signal aperture and one of them undergoes a fade of 10 mmag, for example, the sum of their fluxes undergoes a fade of 5 mmag. Thus, for the large aperture strategy to be better it must exhibit an RMS that is half RMS afforded by a small aperture alternative. Therefore, in the following table I have doubled the large photometry aperture RMS values in the column labeled "RMS2 1 Star" for the large aperture measurements. One final correction has to do with "duty cycle," which is the fraction of time in an observing session when the CCD shutter is open. For the above observations download times were 8 seconds and an additional 4 seconds was spent "settling" the autoguider. Duty cycles are shown in the following table. The final column shows RMS divided by SQRT(DutyCycle), which corresponds to the RMS for each 2 minutes of observing time.

    Strategy                                         RMS2         RMS2 1 Star  DutyCycle    RMS2'

    Sharp focus, small aperture, 30-sec exposures    3.50 mmag    3.50 mmag    0.71        4.14 mmag
    Sharp focus, small aperture, 10-sec exposures    2.06 mmag    2.06 mmag    0.45        3.06 mmag
    Sharp focus, large aperture, 10-sec exposures    3.09 mmag    6.18 mmag    0.45        9.21 mmag
    Defocused,   large aperture, 60-sec exposures    2.02 mmag    4.04 mmag    0.83        4.43 mmag

The WINNER is therefore "sharp focus, small aperture, 10-sec exposures," which provides a precision of 3.06 mmag every 2 minutes for the brightness of HD 80606.

Caveat

It should be kept in mind that I may have defocused too much. Another web page (Defocusing, The General Case) is under construction that suggests that only a small amount of defocusing produces optimum precision for a 9th magnitude star with my hardware. But the counter argument is that for HD 80606 any amount of defocusing will impair the ability to use a small photometry aperture and prevent starlight from HD 80607 from contaminating the photometry of  HD 80606. I believe that this is an over-riding argument, and...

Conclusion

I plan on observing HD 80606 with as sharp a focus as possible!

Since other observers will have different image scales and different atmospheric seeing, if the best quality light curve is to be achieved on February 13/14 it will be necessary for each observer to perform a simple version of what I described on this web page.

WebMaster: B. Gary.  Nothing on this web page is copyrighted. This site opened:  2009.01.30.  Last Update:  2009.02.09