ASTEROID "2004 MN4"
Bruce L. Gary
Links internal to this web page:
Brightness revision
suggestion
Rotation light curve: roation period and
shape ratio
BRIGHTNESS REVISION SUGGESTION
2005.02.05
This web page section shows observations of asteroid "2004 MN4" that have
been calibrated using Tycho stars in the same images used for observing the
asteroid (71 x 47 'arc). All-sky photometry was attempted on several occasions
but the atmospheric extinction was too variable due to scattering from cirrus
clouds. I conclude that this asteroid is ~0.3 magnitude brighter than the
ephemeris value of H = 19.3, i.e., I suggest that H = 19.0. Details are given
below.
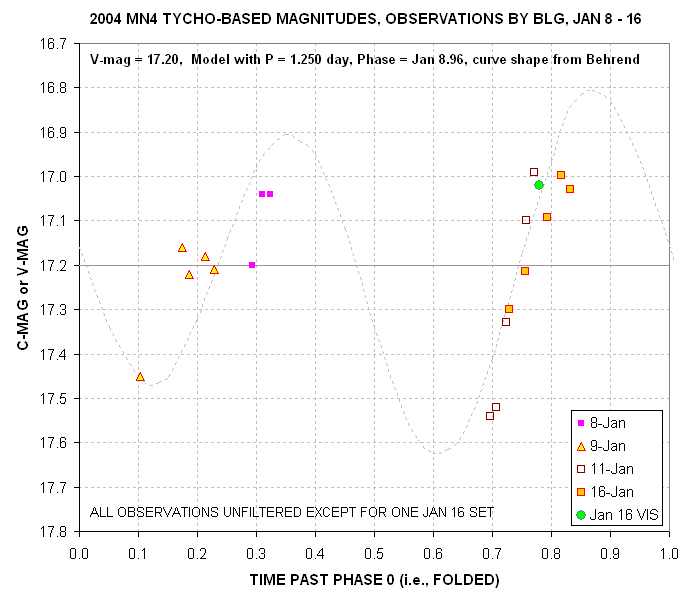
Figure 1. Plot of V-equivalent magnitudes for asteroid "20044
MN4" based on Tycho stars in the same image as the asteroid. No adjustments
have been made to achieve a fit to the model curve, which is based on the
analysis of Dr. Raoul Behrend. The period for this plot is 1.250 days instead
of Behrend's 1.2764 days. The green symbol was made using a V-filter, the
others were unfiltered. Changes in apparent brightness due to changing distance
from Earth have been removed in a way that renders the plot valid for January
16. A known instrumental sensitivity to star color has been applied to the
asteroid using an assumed B-V = 0.80.
For each observing date several Tycho stars were used to establish a
calibration for that night's images. All but one observing set were unfiltered.
Instrumental corrections for unfiltered observations have been established
on many nights with this observing system, and the conversion from star flux
to equivalent V-magnitude, called C-mag, has been found to obey the following
relationship:
C-mag = 21.37 - 2.5 * LOG ( Fv / g ) - Kv * m + 0.30 *
(B-V-0.64) + 0.04 * m * (B-V-0.64),
where
Fv = flux (using a V-filter), g = exposure time [seconds], Kv = zenith extinction
(using a V-filter) [mag/air mass], and m = air mass.
On photometric nights Kv = 0.12 [mag/air mass]. On non-photometric nights
it is higher, and must be established using either Tycho stars or nearby
Landolt stars. For these observations Tycho stars were used for calibration,
and they don't have reliable B-V colors, so I assumed they were typical in
having B-V = 0.64 (the average of all 1259 Landolt stars). The asteroid is
assumed to be redder than typical stars, with B-V = 0.80 +/- 0.05 (as suggested
by Prof. Richard Binzel). One V-filter observation confirms the unfiltered
results taken a few minutes earlier.
The ephemeris currently uses H = 19.3. The magnitudes in the above
plot were adjusted to be valid for January 16, when the ephemeris predicts
a V-magnitude of 17.53. The asteroid appears to be brighter by 0.33 magnitude,
implying that H = 18.97.
The uncertainty on this suggested value for H depends upon the validity of
G. But for now, let us adopt the ephemeris value of G = 0.15. Tthe main uncertainty
would then come from the measurements presented here. The assumed B-V for
the asteroid produces an uncertainty of only 0.004 magnitude (i.e., assuming
B-V = 0.80 +/- 0.05). Another source of uncertainty is the use of Tycho stars
with unknown B-V. Since different Tycho stars were used for each observation
night, and since an average of 3 such stars were averaged, it can be estiamted
that the average of 4 observing nights (12 Tycho stars) introduces an uncertainty
of ~ 0.03 magnitude for the average magnitude of 17.20 (this is based on
an estiamted RMS on B-V for Tycho stars ~ 0.3 magnitude and a star color sensitivity
of 0.30). This is probably the principal systematic error source for the
observations reported here. Another source of uncertainty relates to the
way I used a model rotational light curve for fitting the observations. If,
for example, all observations were at the peak brightness part of such a
light curve then it is obvious that the asteroid's average brightness would
be over-estimated. However, in this case most of the measurements were made
during the rising portion of the light curve. I estimate that this source
of uncertainty is ~0.05 magnitude. Finally, stochastic uncertainty ("noise")
is present, but with SNR typically > 50 for each data group shown in the
above plot this source of uncertainty is estiamted to be < 0.02 magnitude.
I conclude by suggesting that the value for H be revised from 19.3 to 19.0
+/- 0.10, subject to the assumption that G = 0.15.
__________________ The remainder of this web page was created inJanuary,
and was a vehicle for presenting observations by two observer groups
_________________________
ASTEROID "2004 MN4" ROTATION
LIGHT CURVE
Bruce L. Gary and Vishnu V. Reddy
2004.01.15
Introduction
Near Earth Object asteroid "2004 MN4" was discovered last June, was lost,
then was recovered in November. By December 23 the orbit had been established
well enough by NASA's Near Earth Object Program Office at JPL that the NEO
Program Office (Yeomans, Chesly and Choda) posted at MPML that a near Earth
pass in 2029, April 13 had a 1 in 300 chance of Earth impact. Because of
the December 27 re-assessment of a 1 in 37 chance of Earth impact in 2029
an effort was made to refine the orbit with new astrometry observations.
Finally, the Spacewatch telescope at Kitt Peak found an image of the asteroid
(mag 22) taken in March (before the discovery) showing an asteroid position
that was quickly incorporated into orbit calculations that reduced the probability
of Earth impact to near zero. Nevertheless, the pass in 2029 will occur, and
the asteroid may pass close enough (8 Earth radii) to be visible with the
naked eye.
There has been considerable discussion on the MPML about photometry shortcomings,
and the need for better brightness values in order to estimate asteroid size.
Radar observations are planned, and part of the planning requires approximate
maximum Doppler width from the returned echo. Therefore, to assist in radar
planning it is important to obtain brightness measurements that are not only
accurate but precise enough to establish a rotation period.
On January 6 Raoul Behrend reported to the MPML that Yassine Damerdji had
obtained observations at Haute-Provence Observatory from which a rotation
light curve was derived. The light curve solution (by Damerdji et al)
calls for a period of 0.6 +/- 0.4 day (14.4 +/- 9.6 hours) and an amplitude
of 0.2 mag (half of peak-to-peak variation). The light curve can be found
at http://obswww.unige.ch/~behrend/page5cou.html#04m04n.
January 8 and 9 Light Curve Observations
On UT dates January 8, 9 and 11 Vishnu V. Reddy (University of North
Dakota graduate student) and Ken Archer (Ironwood Observatory, Hawaii) used
the 10-inch Takahashi Baker-RC f/5 telesope and SBIG ST8 CCD of the Ironwood
Observatory, Hawaii (F60) to observe 2004 MN4. These observations were conducted
remotely from North Dakota by VVR using the Share My Sky program.
On January 8, 9 and 11 Bruce Gary observed "MN4" using the Hereford Arizona
Observatory (G95) Celestron 14-inch SCT and SBIG ST-8XE CCD. The January
9 and 11 observations were made through cirrus clouds with extinction variations
of 0.6 magnitude. Our data have been combined and are shown in the following
figure.
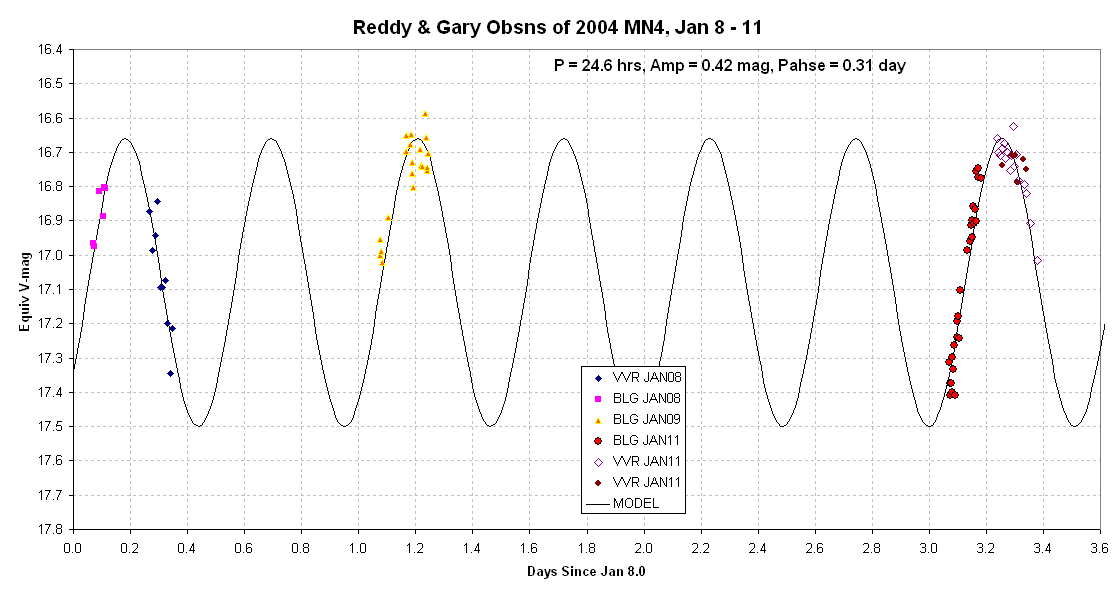
Figure 1. Equivalent V-magnitudes (from unfiltered observations)
during a 4-day observing interval. The magnitude scale is set by a January
11 image (by BLG) with several Tycho stars in the same FOV as the asteroid.
All other data sets were zero-shifted to agree with the BLG Jan 11 data.
The BLG observations (at Hereford Arizona Observatory, G95) were made by
Bruce L. Gary with a 14-inch Celestron and SBIG ST-8XE CCD, and were processed
by median combining sets of three 60-second exposures using the asteroid
for alignment. The VVR observations (at Ironwood Observatory, Hawaii,
F60) are 5-point averages of 60-second exposures.
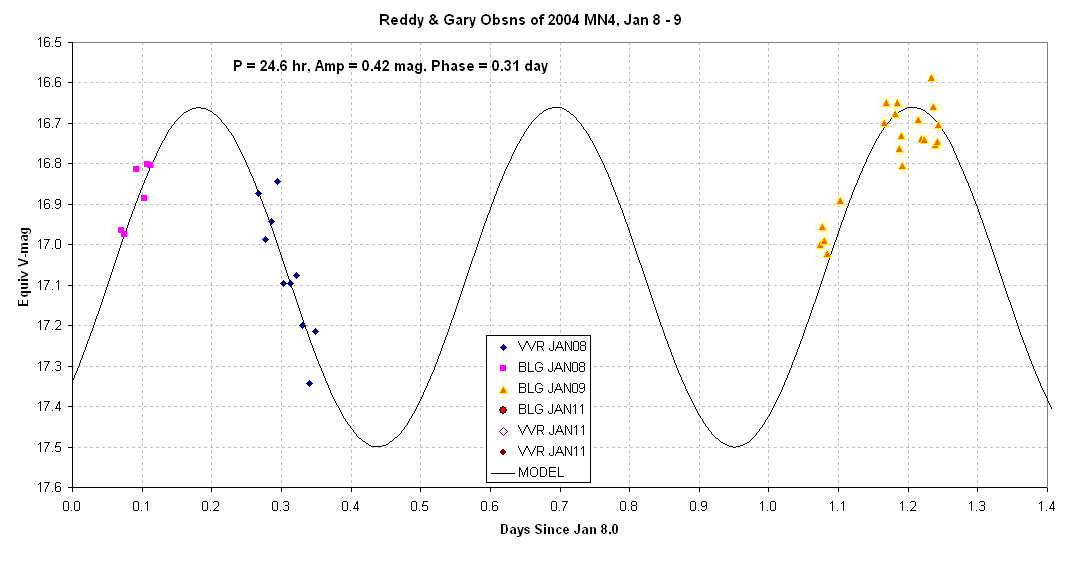
Figure 2. Same as previous figure except showing only the
Jan 8 and 9 observations.
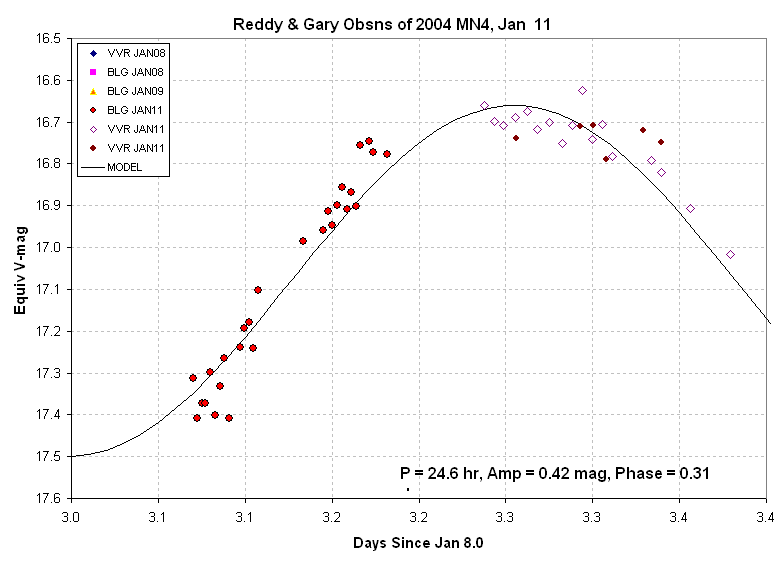
Figure 3. Same as first figure except showing only the Jan
11 observations.
The fitted sinusoidal model solution has two periods per rotation period.
The rotation period from this data (alone) is estimated to be ~24.6 hours.
The amplitude (half of peak-to-peak) is 0.42 magnitude. I hesitate to give
SE uncertainties since I've been wrong about this several times in the past
week.
Note that using a sinusoid is just a first approximation for fitting an asteroid
rotation light curve. Shape matters, and all real asteroid shapes produce
light curve shapes that depart from sinusoidal.
Analysis Procedure Used by BLG
The procedure used by BLG for reducing images to asteroid magnitudes involves
two analysis phases. The first phase transfers magnitudes from Tycho stars
in the FOV to secondary stars near the asteroid's path. The second phase
uses these secondary calibration stars to determine the magnitude of the
asteroid in sets of 3 median combined images.
The first phase consists of the following: calibrate raw images (dark and
flat), median combine neighbor sets of 3 images using stars for alignment
(MCs images), median combine the same 3 images using the asteroid for alignment
(MCa images), read intensity of MCs Tycho stars for several images, determine
extinction and zero-shift parameter in a spreadsheet, use these extinction
and zero-shift values to determine magnitude of stars near the asteroid's
path, adopt the average magnitude for these secondary calibration stars for
use as reference stars.
The second phase consists of the following: perofrm two median combines
for each set of 3 images, one aligned using the asteroid and the other aligned
using the stars. Intensity readings are made of the (three) secondary reference
stars on the MCs images, and intensity readings are made of the asteroid
on the MCa iamges (placing the photometry pattern so as to minimize the influence
of background level biases). These intensity readings are entered in a spreadsheet
that has been prepared for this specific analysis procedure. A block of cells
for each image is used to establish an extinction for that image in a way
that produces reference star magnitudes that agree (on average) with those
adopted in the first phase of analysis (taking into account the image's air
mass). The zero-shift and extinction values allow for a conversion of the
asteroid's intensity to be converted to a magnitude.
This analysis procedure may be unique in the way it allows for asteroid motion
between images. Notice that all intensity readings are done with images that
have cosmic ray defects removed and SNR enhanced by median combining. Also
note that the median combining is performed separately for images intended
for reference star intensity readigns and asteroid intensity readings. This
median combining using the asteroid for alignment requires that the asteroid
can be seen in each image, which places a practical limit on how faint the
asteroid can be for using the procedure. For an asteroid moving at the rate
of 2004 MN4 when these observations were made (~3.8 "arc/minute) the longest
exposure time for avoiding oval asteroid source functions was 60 seconds
for my image scale of 2.8 "arc/pixel. For a 14-inch aperture telscope and
50-second exposures the faintest asteroid for which this method can work
is about V-mag = 18. For a larger telescope, such as 32-inch aperture, the
limiting asteroid magnitude ~ 20 (unless sophisticated techniques are used
to anticipate the asteroid's pixel location.)
Raoul Behrend's Analysis
Raoul Behrend has combined this data with measurements by Yassine Damerdji
and the early data by VVR and BLG to produce a new period of 22.97 +/- 0.14
hours. The amplitude for the solution of the data in the previous figure
(0.27 +/- 0.10 magnitude) is compatible with the 0.3 +/- ~0.05 magnitude
amplitude given by Behrend using the combined data. The combined data calculated
by Raoul Behrend can be found at Raoul Behrend's rotation
light curves . The light curve solution at this site is likely to change
as new observations are added to the analysis.
Here's my version of Raoul Behrend's rotational light curve period of 1.2733
+/- 0.0026 days, fitted to the data available to me (VVR and BLG).
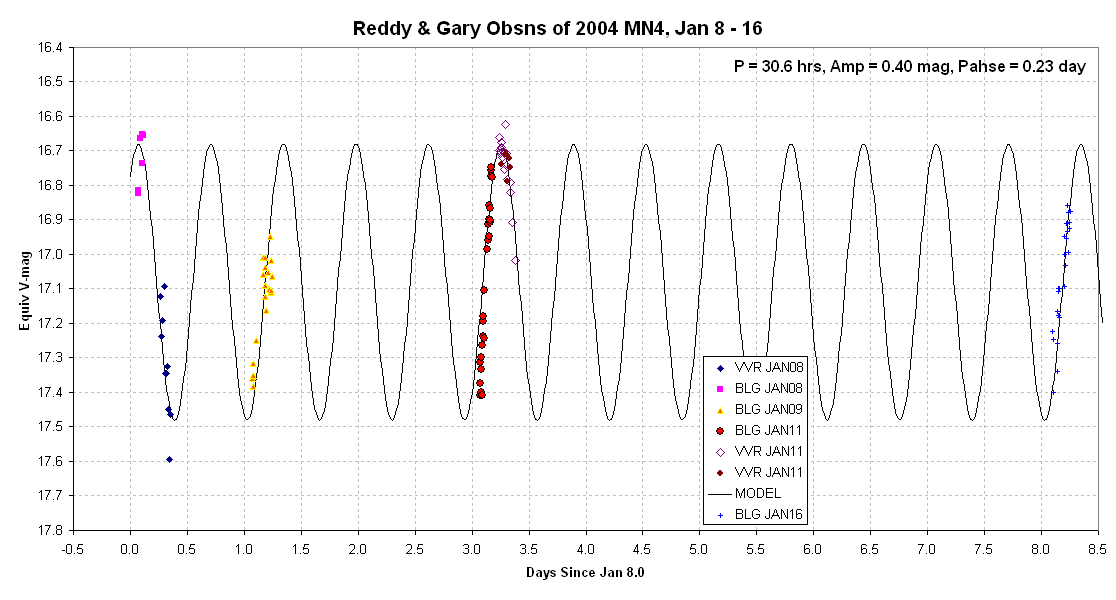
Figure 4. Adopting Raoul Behrend'srotation period solution of
1.2733 days (30.6 hours) and allowing for zero-offset adjustemtns, it is
possible to achieve an acceptable fit to the VVR and BLG observations.

___________________________________________________________
This site opened: January 9, 2005. Last Update: February 5, 2005




